√100以上 if u(x y)=x^2 y^2 2x-3xy then 201764

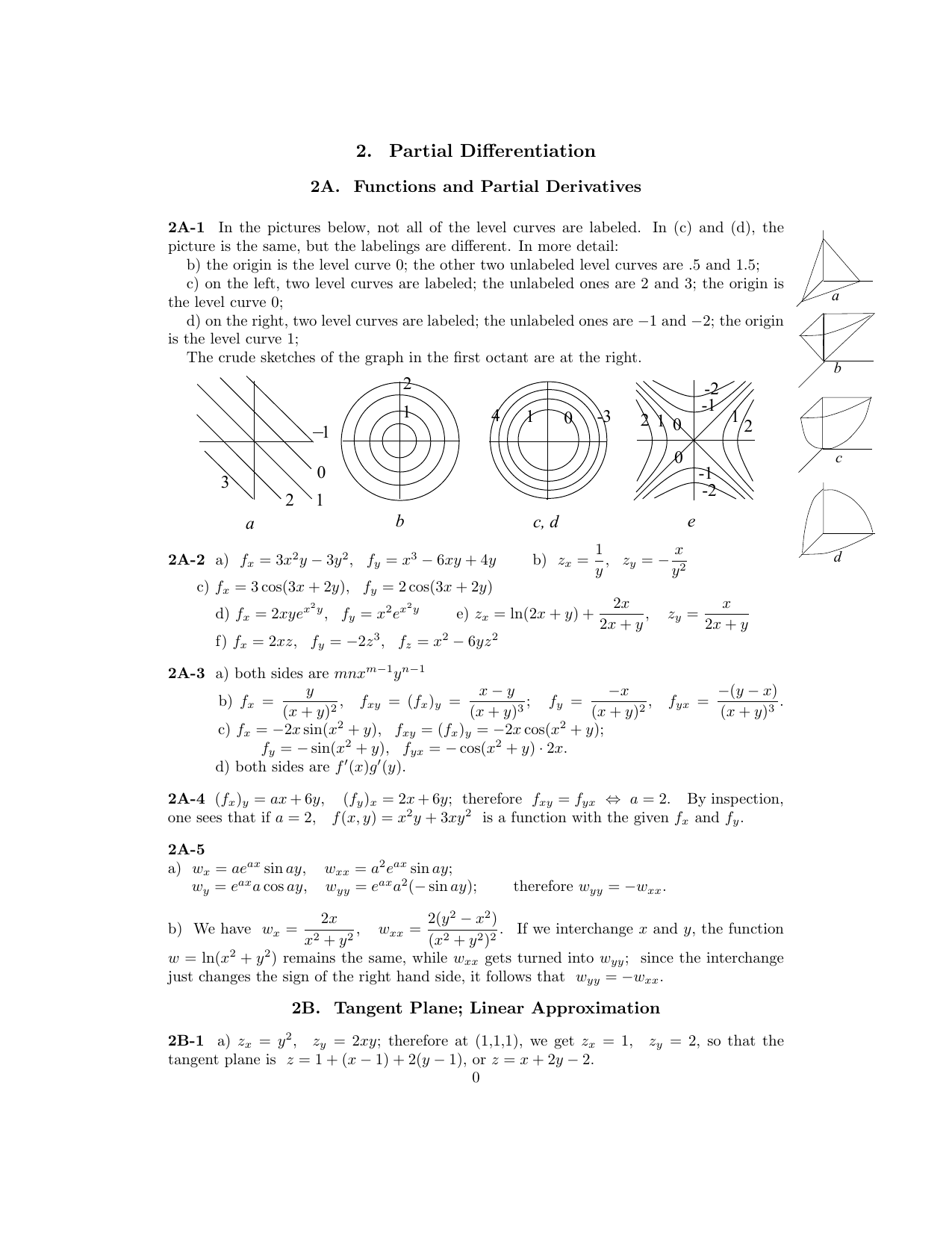
If (x y )sin u = x2y2, then x ( partial u/ partial x) y ( Q If (x y )sin u = x 2 y 2, then x ∂ u ∂ x y ∂ u ∂ y =X^2x6=0x3\gt 2x1 (x5)(x5)\gt 0;26/7/19 If 2x^2 – 3xy y^2 x 2y – 8 = 0, then find dy/dx ← Prev QuestionNext Question → 0votes 212kviews askedin Mathematicsby Devanshi(674kpoints) editedby faiz If 2x2– 3xy y2 x 2y – 8 = 0, then find dy/dx class12 Share It On

2

If u(x y)=x^2 y^2 2x-3xy then

If u(x y)=x^2 y^2 2x-3xy then-If u = ex^2y^2, then Login Study Materials NCERT Solutions NCERT Solutions For Class 12 NCERT Solutions For Class 12 Physics;Given (xy) sin u = x 2 y 2 sin u = x 2 y 2 / (xy) = x 4 (y 2 /x 2 )/x (1 (y/x)) = x 3 (y/x) 2 / (1 (y/x)) = x 3 f (y/x) This is a homogeneous function of degree 3 So by Euler's theorem x∂z/∂x y ∂z/∂y = nz Here n = 3

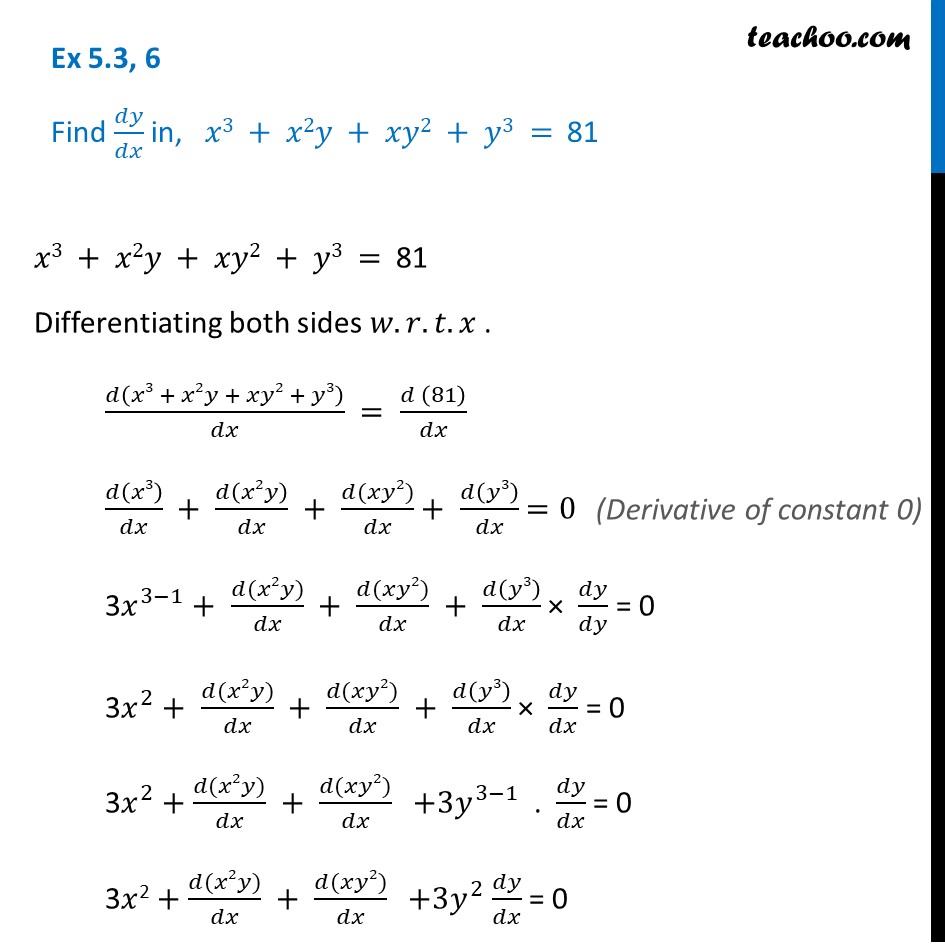
Ex 5 3 6 Find Dy Dx In X3 X2y Xy2 Y3 81 Cbse

Answer Given, u =tan−1( xyx2 y2 ) ⇒ tanu = (xy)x2 y2 On differentiating both the sides wrt x and y respectively, we get sec2udxduIf u= log(x2 y2), then by the chain rule u x= 2x x 2 y) u xx= (x2 y2)(2) (2x)(2x) (x 2 y) = 2y2 2x2 (x y2)2;If u =log(x2 y2 z2), verify ∂ x∂ y∂ 2u = ∂ x∂ y∂ 2u If

PARTIAL DERIVATIVE LINKSImplicit differentiation Partial derivative (i) y cos x = x^2y^2 (ii) e^z = xyz https//youtube/N6TLvbDCOUkLagrange's Multip4/4/18 Explanation We have u = (1 − 2xy y2)−1 2 = 1 2(1 − 2xy y2) and we seek to validate that f satisfies the Partial differential Equation x ∂u ∂x − y ∂u ∂y = y2u3 (In other words we are validating that a solution to the given PDE is u )X 3 y − x 2 y − 2 x y = 0 https//wwwtigeralgebracom/drill/x~3yx~2y2xy=0/ x3yx2y2xy=0 Four solutions were found x = 2 x = 1 y = 0 x = 0 Step by step solution Step 1 Step 2 Pulling out like terms 21 Pull out like factors x3y x2y

Consider 2x^ {2}3xy2y^ {2}2x11y12 as a polynomial over variable x Find one factor of the form kx^ {m}n, where kx^ {m} divides the monomial with the highest power 2x^ {2} and n divides the constant factor 2y^ {2}11y12 One such factor is 2xy4\\ $ Differentiating u partially w r t r and θ,State Euler'S Theorem on Homogeneous Function of Two Variables and If U = X Y X 2 Y 2 Then Evaluate X ∂ U ∂ X Y ∂ U ∂ Y University of Mumbai BE Biomedical Engineering Semester 1 (FE First Year) Question Papers 141 Important Solutions 526 Question Bank Solutions 528

How To Solve The Differential Equation 2y 3xy 2 Dx X 2x 2y Dy 0 Quora

Exercises 1 Show That X Y Is Harmonic In Some Chegg Com

Find dy/dx x^2y^2=2xy Differentiate both sides of the equation Differentiate the left side of the equation Tap for more steps Differentiate Tap for more steps By the Sum Rule, the derivative of with respect to is Differentiate using the Power Rule which states that is whereGráfico y=2x y = 2x y = 2 x Use la ecuación general de la recta para hallar la pendiente y la intersección en y Toca para ver más pasos La ecuación general de la recta es y = m x b y = m x b, donde m m es la pendiente y b b es la intersección en y y = m x b y = m x b Encuentre los valores de m m y b b usando la forma y = mCalculus Find dy/dx x2xyy^2=2 x 2xy y2 = 2 x 2 x y − y 2 = 2 Differentiate both sides of the equation d dx (x 2xy y2) = d dx(2) d d x ( x 2 x y − y 2) = d d x ( 2) Differentiate the left side of the equation Tap for more steps

If 2x 2 3xy Y 2 X 2y 8 0 Then Dy Dx Youtube

2 Partial Differentiation

V x = u y = (2y) = 2y;Question 2 If U(x, Y) = 3x²y 2x2 Y3 – 2y Then A) Check U(x,y) Is Harmonic Or Not B) Find The Analytical Function F (2) This question hasn't been answered yet Ask an expert Show transcribed image text Expert Answer Previous question Next question Transcribed Image TextIf X and Y are two independent variables, then Cov (X,Y) = 0 But the Converse need not be true 2Cov (aX, bY) = ab Cov (X,Y) 3Cov (X a,Y b) = Cov (X,Y) 49Show that Cov(aXb,cYd)=acCov(X,Y) Answer Take U= aXb and V= cYd Then E(U)=aE(X)b and E(V)= cE(Y)d UE(U)= aXE(X) and VE(V)=cYE(Y)

7 F X Y X Y Subject To X 2 Xy Y 2 Chegg Com

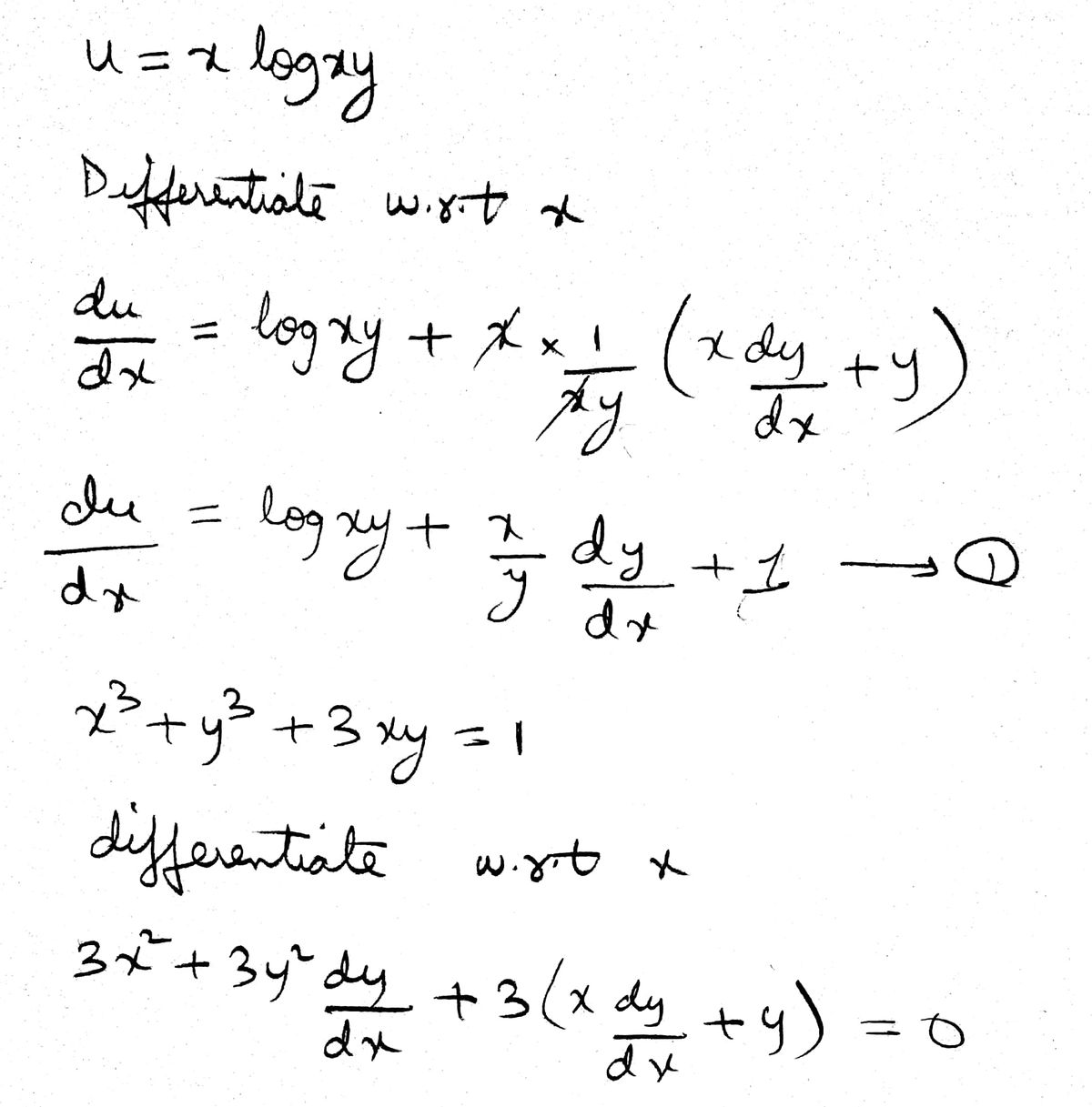
Answered If U X Log Xy Where X3 Y3 3xy 1 Find Bartleby

Assertion (A) If u = x 2 y 2 z 2 , then ∂ x 2 ∂ 2 u ∂ y 2 ∂ 2 u ∂ z 2 ∂ 2 u = u 1 Reason(R) If u = f ( r ) and r 2 = x 2 y 2 z 2 , then ∑ ∂ x 2 ∂ 2 u = f ′ ′ ( r ) r 2 f ′ ( r )NCERT Solutions For Class 12 Maths;28/1/21 Solution u = sin 1 ( (x 2 y 2 )/ (xy)) sin u = (x 2 y 2 )/ (xy)) = x 2 (1y 2 /x 2 )/x (1y/x) = x (1y 2 /x 2 )/ (1y/x) = x f (y/x) This is a homogeneous function of degree 1 So by Euler's theorem x∂z/∂x y ∂z/∂y = nz

If U X 2tan 1 Y X Y 2tan 1 X Y Prove That 2u Y X X 2 Y 2 X 2 Y 2 Sarthaks Econnect Largest Online Education Community

2

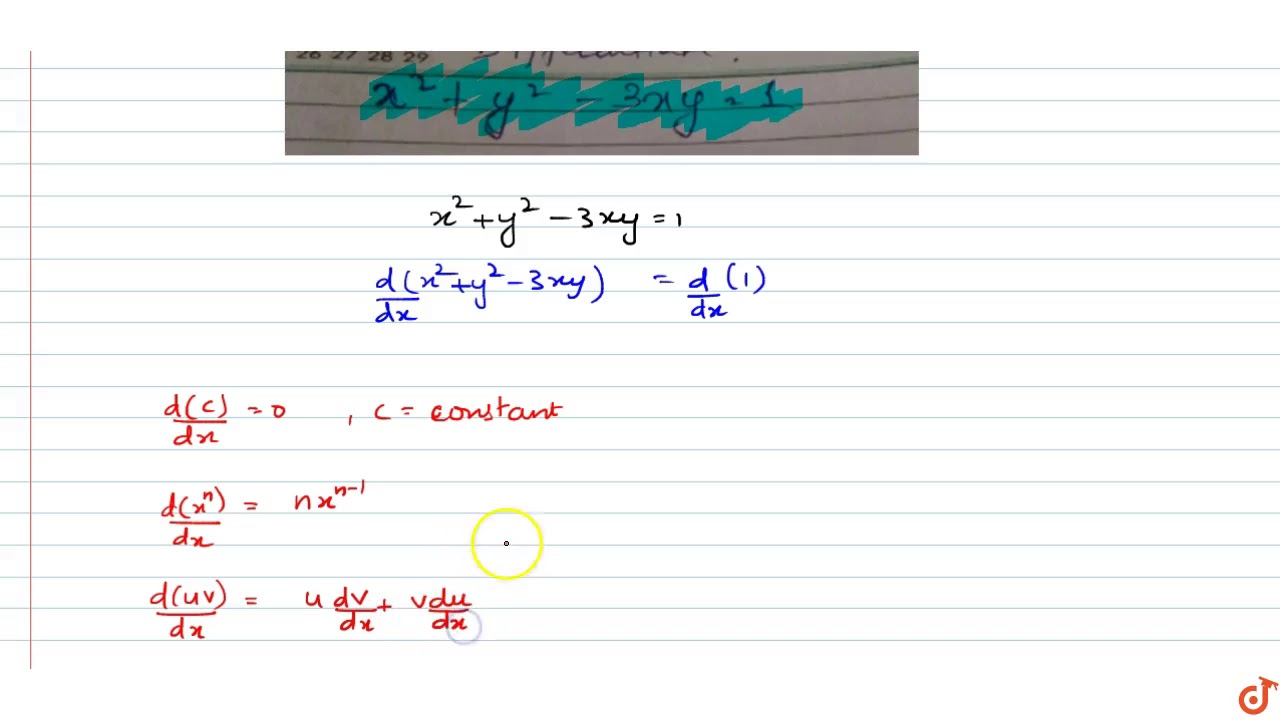
31/3/15 Correction (after missing a sign) As kobe pointed out, the original DE is $$ (x^2y^2)y'2xy=0, $$ which as equation for a vector field reads $$ (x^2y^2)\,dy2xy\,dx=0\iff Im(\bar z^2\,dz)=0\text{ with } z=xiy $$ From the complex interpretation it is directly visible that this is not integrable, for that it would have to be an expression $Im(f(z)\,dz)$Concept If two functions u and v satisfy CauchyRiemann equations, then they are said to be harmonic conjugates with respect to each other CauchyRiemann equations are v y = u x v x = u y Calculation Given u = x 2 – y 2, let v be the harmonic conjugate By CauchyRiemann equations, v y = u x = 2x;Calculus Solve for y x^23xyy^2=1 x2 − 3xy y2 = 1 x 2 3 x y y 2 = 1 Move 1 1 to the left side of the equation by subtracting it from both sides x2 − 3xyy2 − 1 = 0 x 2 3 x y y 2 1 = 0 Use the quadratic formula to find the solutions −b±√b2 −4(ac) 2a b ± b 2 4 ( a c) 2 a Substitute the values a = 1 a = 1, b

Assignment No

2

1(r)es, x y = 2c 2(r)e s, so x2 y2 = (xy)(x y) = 4c 1(r)c 2(r), ie is a constant along the projected characteristic curves In other words, the projected characteristic curves are x 2 y = C, C a constant, and the solution is a function that is constant along these One has toSubstitute (x−1)2 − 1 ( x 1) 2 1 for x2 −2x x 2 2 x in the equation x2 − 2xy2 = 0 x 2 2 x y 2 = 0 Move −1 1 to the right side of the equation by adding 1 1 to both sides Add 0 0 and 1 1 This is the form of a circle Use this form to determine the center and radius of the circleNCERT Solutions For Class 12 Biology;

X 3 3xy 2 Dx Y 3 3x 2y Dy Youtube

If X Y X Y X Y 3xy Then What Is X And Y Mathematics Solutions

Math 9 Assignment 2 — Solutions 1 Let R = ln(u2 v2 w2), u = x 2y, v = 2x − y, and w = 2xyUse the Chain Rule to find ∂R ∂x and ∂R ∂y when x = y = 1 Solution The Chain Rule gives ∂R ∂xUsa la forma a x 2 b x c a x 2 b x c para encontrar los valores de a a, b b y c c a = 1, b = − 2, c = 0 a = 1, b = 2, c = 0 Considera la forma canónica de una parábola a ( x d) 2 e a ( x d) 2 e Reemplazar los valores de a a y de b b en la fórmula d = b 2 a d = b 2 a d = − 2 2 ( 1) d = 2 2 ( 1) Simplificar el lado derechoWe have dv = v x dx v y dy ⇒ dv = 2y dx 2x dy =

2

Partial Differentiation If U X 2 Y 2 Z 2 X E T Y E Tsint Z E Tcost Prove That Du Dt 4e T Youtube

2/8/19 If u = log(x^2 y^2)/xy then x(∂u/∂x) y(∂u/∂y) is (a) 0 (b) u (c) 2u asked in Differentials and Partial Derivatives by RamanKumar ( 499k points)Given sinu= xyx2 y2 Differentiating wrt x, we get,cosudxdu = (xy)22x(xy)−(x2 y2) cosudydu = (xy)22y(xy)−(x2 y2) xdxdu ydydu = secu( (xy)2y3 y2xx2yx3 )=Verificar\\tan^2(x)\sin^2(x)=\tan^2(x)\sin^2(x) \frac{d}{dx}(\frac{3x9}{2x}) (\sin^2(\theta))' \sin(1) \lim _{x\to 0}(x\ln (x)) \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x

2

Find An Analytic Function Whose Real Part Is U X 3 3xy 2 3x 2 3y 2 3y 2 1 Sarthaks Econnect Largest Online Education Community

Given, \(u = log\left( {\frac{{{x^2} {y^2}}}{{x y}}} \right)\) \(z = \frac{{{x^2} {y^2}}}{{x y}}\) z is a homogenous function of x & y with a degree 1 Now, z = e u Thus, by Euler's theorem \(x\frac{{\partial u}}{{\partial x}} y\frac{{\partial u}}{{\partial y}} = \frac{{{e^u}}}{{{e^u}}}\) \(x\frac{{\partial u}}{{\partial x}} y\frac{{\partial u}}{{\partial y}} = 1\) Download Question WithThis is easy if you don't insist on using Lagrange multipliers The normal to the ellipse at the point (x, y, z) is ∇ (x 2 y 2 4 z 2) = (2 x, 2 y, 8 z) At minimum or maximum distance to the plane,Given The function is given by, u = F ( y−x xy, z−x xz) u = F ( y − x x y, z − x x z) The condition is given by, x2,y2,z2 ⋅∇u = 0 x 2, y 2, z 2 ⋅ ∇ u = 0 Show that the condition

Ex 5 3 6 Find Dy Dx In X3 X2y Xy2 Y3 81 Cbse

If X 2 And X Square Y Square 3xy 5 Then Find Y Brainly In

28/8/ If U(x,y,z) = (x^2 y^2)/xy 3z^2y, find ∂U/∂x, ∂U/∂y and ∂U/∂z asked in Differentials and Partial Derivatives by RamanKumar ( 499k points) differentialsNCERT Solutions For Class 12 Chemistry;\\ v=x^2y^2=r^2 cos^2 θr^2 sin^2 θ=r^2 (cos^2 θsin^2 θ)=r^2 cos⁡2θ \\ \;

If X Y 2 Then What Is The Value Of X Y 6xy Quora

Solve The Equation X 2 3xy Y 2 Dx X 2dy 0 Given That Y 0 And X 1

Y = x – x 2 Squaring both sides y 2 = (xx 2) 2 y 2 = x 22x 3 x 4 Differentiate wrt x 2 dy 2 /dx 2 = (d/dx 2)( x 2 (x 2) 2 – 2(x 2) 3/2) = 1 2x 2 – 2(3/2)x =R x as boundaries the curves x2 −y2 = 1, x2 −y2 = 4, y = 0, y = x/2 Solution Since the boundaries of the region are contour curves of x2 −y2 and y/x , and the integrand is y/x, this suggests making the change of variable (23) u = x 2 −y 2 , v = y x We will try to get through without solving these backwards for x, y in terms of u, v Find answer in image to clear your doubt instantly If is equal to 1 (b) 2 (c) (d) none of these 102k 47k 2 If (A) 36 (B) (D) 21k

If U Log X 2 Y 2 Xy Then X U X Y U Y Is A 0 B U C 2u Sarthaks Econnect Largest Online Education Community

Chapter 7 Calculus Of Several Variables

The given information is u = log(x2 y2x4 y4 )Taking partial differentiation wrt to x and y one at a time we get,⇒ ∂ x∂ u = x4 y4x2 y2 (x2 y2)24x3(x2 y2)−2x(x4 y4) ⇒ ∂ x∂ u = (x4 y4)(x2 y2)4x5 4x3y2 −2x5 −2xy4 ⇒ ∂ y∂ u = x4 y4x2 y2 (x2 y2)24y3(x2 y2)−2y(x4 y4) ⇒ ∂ y∂ u = (x4 y4)(x2 y2)4y5 4x2y3 −2y5Now you have to differentiate this equation 2 times From 1st differentiation you will get => 2× (s3t)×12×2× (2st) ie 2× (s3t)4× (2st) now again differentiate with respect to "s" , since 't' is a constant term here it will become "0" so you have 24×2 ieIf u=sin1((x^2y^2)/(xy)) then show that x(du/dx)y(du/dy)=tan u MATHEMATICS1 question answer collection

Worked Example Implicit Differentiation Video Khan Academy

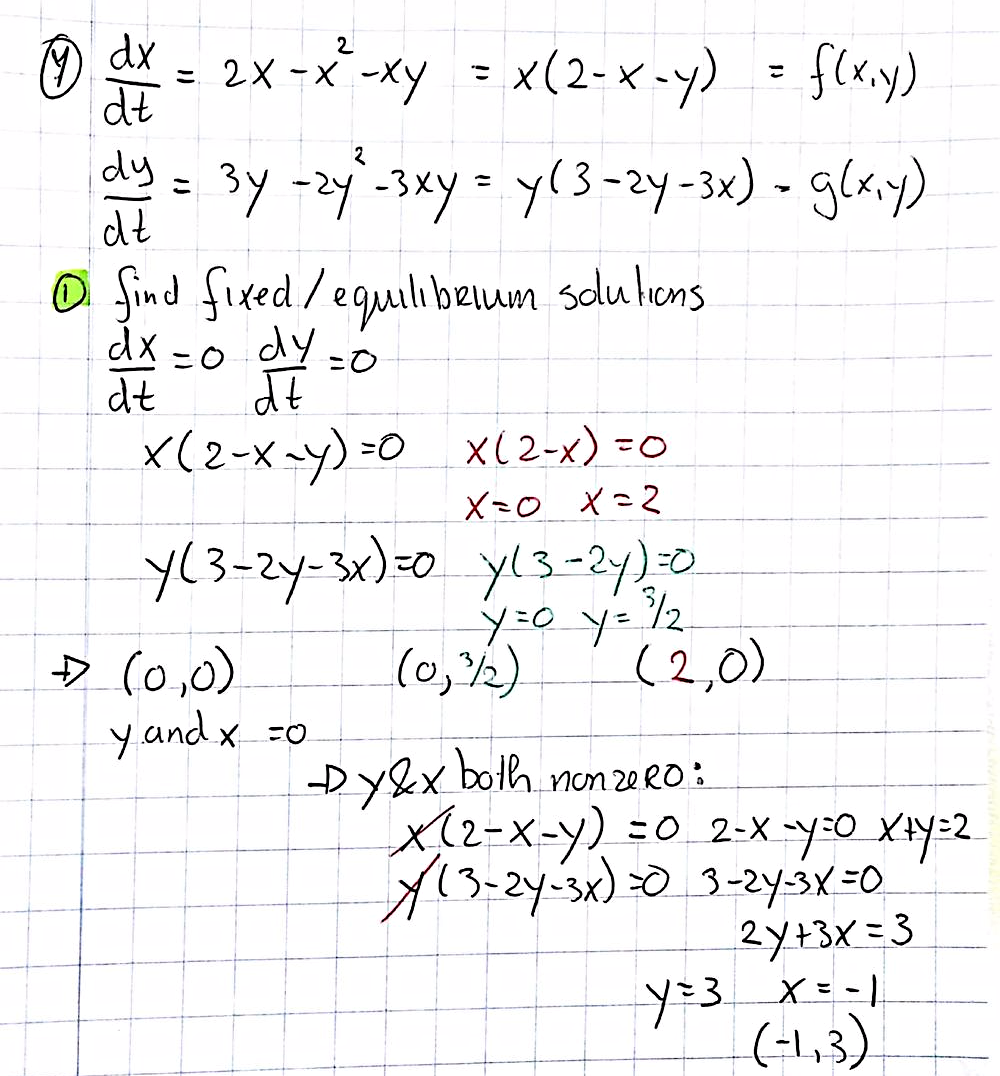
A Find All The Critical Points Equilibrium Solutions B Use A Computer To Draw A Direction Field And Phase Portrait For The System C From The Plot S In Part B Determine Whether Each Critical Point

81k views If u = x 2 − y 2, v = 2 x y a n d z = f ( u, v) prove the following written 49 years ago by shailymishra30 ♦ 330 modified 14 months ago by sanketshingote ♦ 570 ( ∂ z ∂ x) 2 ( ∂ z ∂ y) 2 = 4 u 2 v 2 ( ∂ z ∂ u) 2 ( ∂ z ∂ v) 2 partial differentiation ADD COMMENTOr,x^yy^x=x^3y^39xy again x=3y so x^3y^39xy= (3y)^3y^39 (3y)y=2727y9y^2y^3y^39y^227=5427y Now we got 54 27y=27, or 27y=27,y=1 Then x=31=2 The values of x& y only satisfy xy=3 and doen't satisfy x^yy^x=27 So there is no solution for the values of x & y 305 views Answer requested byAnd by the symmetry of uin xand y, u yy= 2x2 22y (x2 y2)2 Clearly then u xx u yy= 0 in this case If u= arctan(y=x), then by the chain rule again u x= 1 1 (y x) 2 y x 2 = 2y x y 2) u xx= (x y2)(0) ( y)(2x) (x y2) = 2xy (x2 y2)2 Likewise u y= 1 1 (y x) 2 1 x = x x2 y 2) u yy= (x2 y2)(0) (x)(2y) (x 2 y2) = 2xy (x y)2

2 Partial Differentiation

Jacobians New

Factor 2x^23xyy^2 2x2 3xy y2 2 x 2 3 x y y 2 For a polynomial of the form ax2 bx c a x 2 b x c, rewrite the middle term as a sum of two terms whose product is a⋅c = 2⋅1 = 2 a ⋅ c = 2 ⋅ 1 = 2 and whose sum is b = 3 b = 3 Tap for more steps Reorder terms 2 x 2 y 2 3 x y 2 x 2 y 2 3 x y Reorder y 2 y 2 and 311/9/18 Stack Exchange network consists of 177 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers Visit Stack Exchange$\begingroup$ Any solution of your PDE is radially symmetric, ie of the form $u(x,y)=f(r)$ where $r=\sqrt{x^2y^2}$, and radial function can not satisfy the condition $u(x,0)=1$ $\endgroup$ – Mercy King Jun 28 '13 at 948

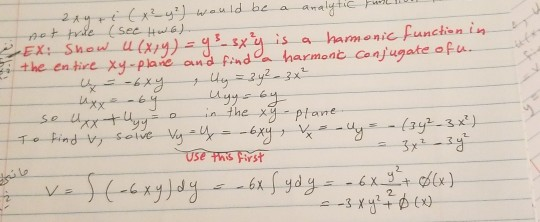
Exercises 1 Show That U X Y Is Harmonic In Some Chegg Com

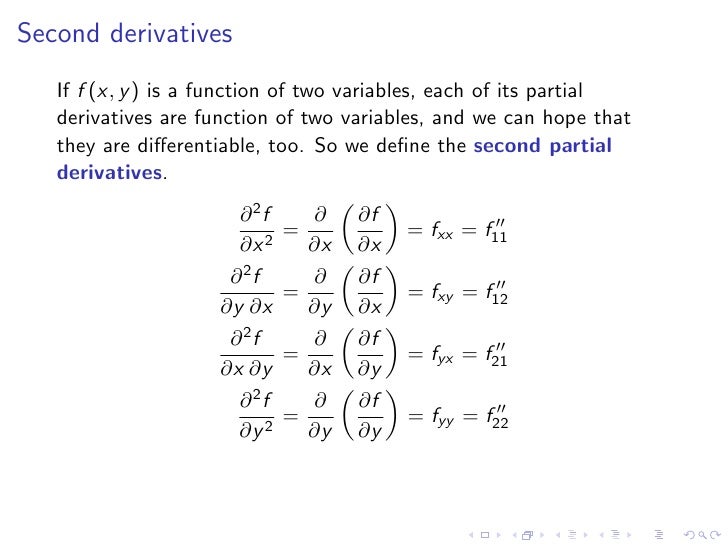
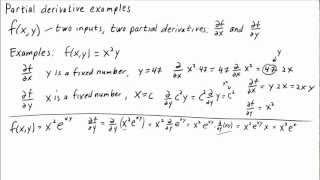
Lesson 19 Partial Derivatives

All equations of the form a x 2 b x c = 0 can be solved using the quadratic formula 2 a − b ± b 2 − 4 a c The quadratic formula gives two solutions, one when ± is addition and one when it is subtraction yx^ {2}y^ {2}xu=0 y x 2 y 2 x − u = 0 This equation isYu x = xu y (3) yu x xu y = 0 (4) x 2 u y y 2 u x = 0$u=2xy=2(r cos⁡θ )(r sin⁡θ )=2r^2 cos⁡θ sin⁡θ=r^2 sin⁡2θ \\ \;

Ex 9 5 15 Class 12 Find Solution 2xy Y 2 2x 2 Dy Dx 0 When

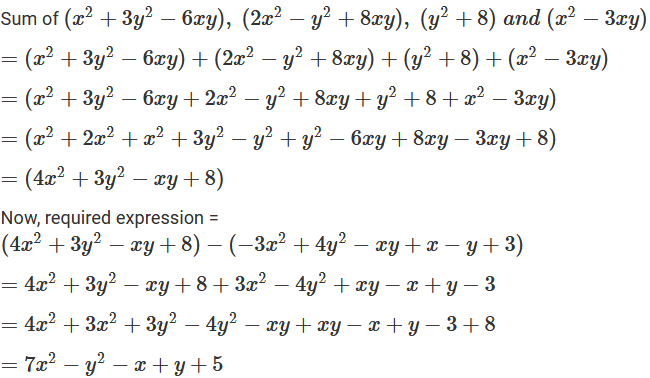
R D Sharma Solutions Class 7 Math Chapter 7 Algebraic Expressions Exercise 7 2

2

If U X Y Z Xy 2z 3 X Sin T Y Cos T Z 1 E 2t Find Du Dt Sarthaks Econnect Largest Online Education Community

Prove That X2 Y2 C X2 Y2 2 Is The General Solution Of The Differential Equation X3 3xy2 Dx Y3 3x2y Dy Where C Is A Parameter From Class

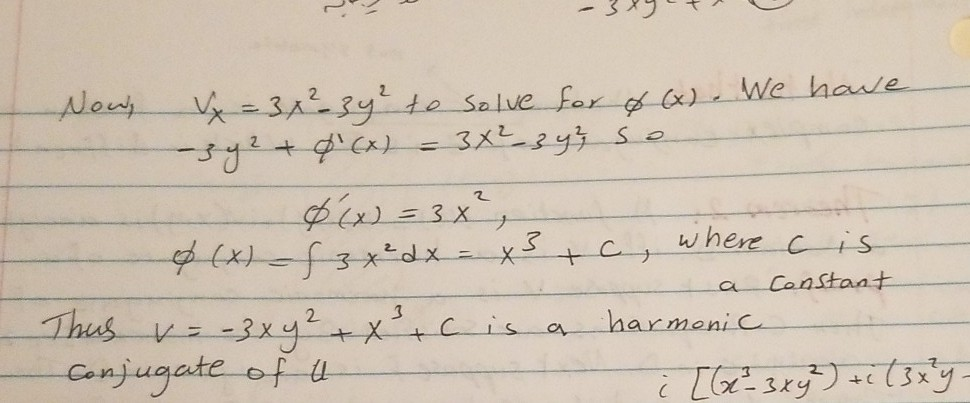
Exercises 1 Show That U X Y Is Harmonic In Some Chegg Com

If X 2 Y 2 3xy Then Choose The Correct Answer Of 2log X

Systems Of Equations With Elimination 4x 2y 5 2x Y 2 5 Video Khan Academy

What Is The Derivative Of 3xy

Partial Derivative Examples Math Insight

Show That The Equation Tex X 2 3xy 2 Y 2 2x 3y 35 0 Tex For Every Real Value Brainly In

Differentiate X 2 Y 2 3xy 1 Youtube

Vector Analysis By Alimkanwalimtinaa Issuu

2

1 Find And Sketch The Domain Of The Following Chegg Com

2

If W X Y Z X 2 Y 2 Z 2 X E T Y E T Sin T And Z E T Cos T Find Dw Dt Sarthaks Econnect Largest Online Education Community

2

Partial Differentiation

If X 3 2x 2y 2 5x Y 5 0 And Y 1 1 Then A Y Prime 1 4

If Math X Y 2 Text And X 2 Y 2 2 Math What Is The Value Of Math Xy Math Could You Break It Down For Me Quora

T Qmo3 If Uleft X1yright 4x Y See How To Solve It At Qanda

Verify That The Differential Equation 3xy Y 2 Chegg Com

Variablecomplejaysusaplicaciones 7maedicion Churchill Solucionario Co

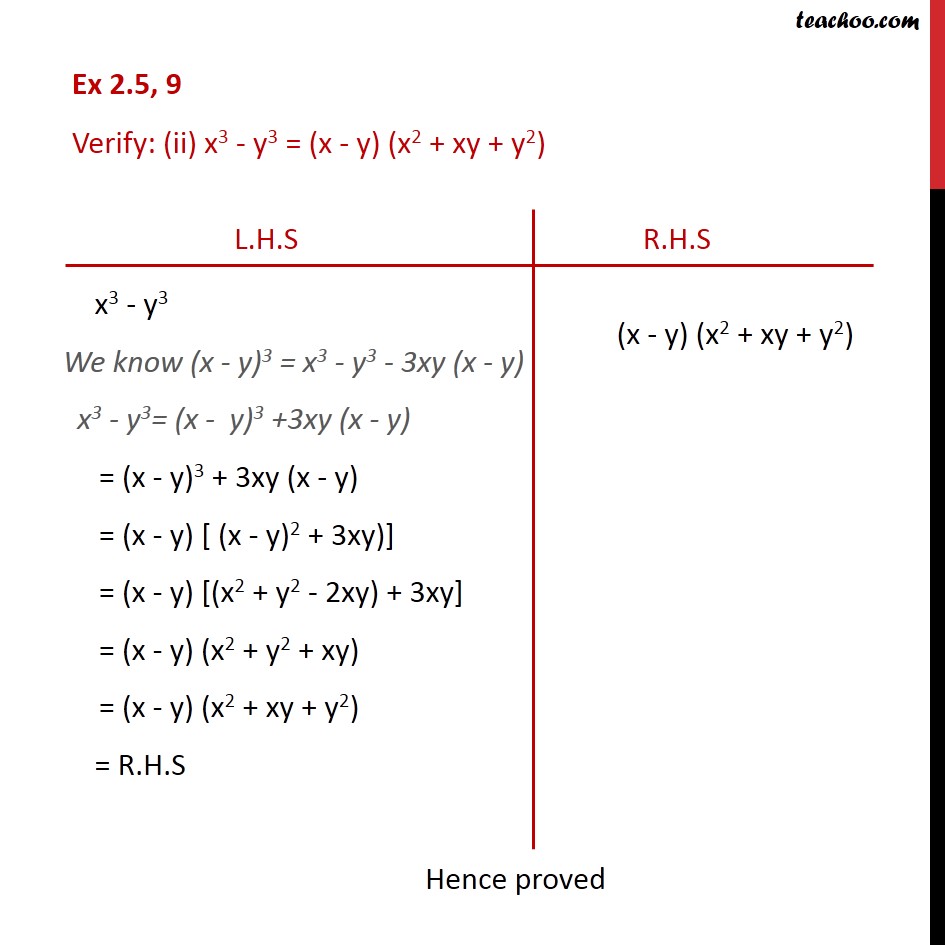
Ex 2 5 9 Verify I X3 Y3 X Y X2 Xy Y2 Ex 2 5

14 2 Limits And Continuity

2

11 Business Math Stats Vol 2 Em Pages 51 100 Flip Pdf Download Fliphtml5

4 Partial Derivatives And Their Applications Derivative Variable Mathematics

3 8 Implicit Differentiation Calculus Volume 1

2

Find The Critical Points Of The Following Functions Use The Second Derivative Test To Determine If Possible Whether Each Critical Point Corresponds To A Local Maximum Local Minimum Or Saddle Point Confirm

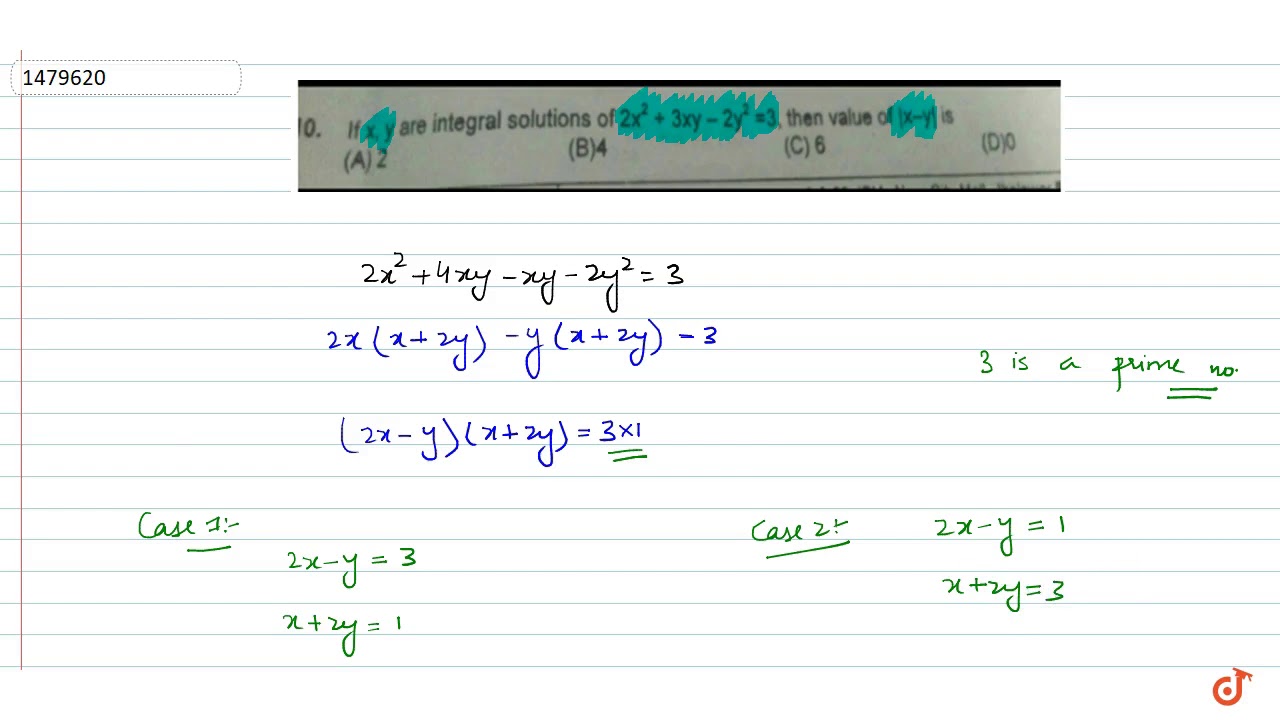
If X Y Are Integral Solutions Of 2x 2 3xy 2y 2 3 Then Value Of X Y Is Youtube

1

Second Partials Test For F X Y X 3 3xy Y 3 Youtube

Rd Sharma Solutions For Class 8 Chapter 6 Algebraic Expressions And Identities Download Free Pdf

What Is The Derivative Of 3xy

2

If 2x 2 3xy Y 2 X 2y 8 0 Then Dy Dx

Solved 4 6 Find The Directional Derivative Of F A

Multivariate Functions And Partial Derivatives Sage Research Methods

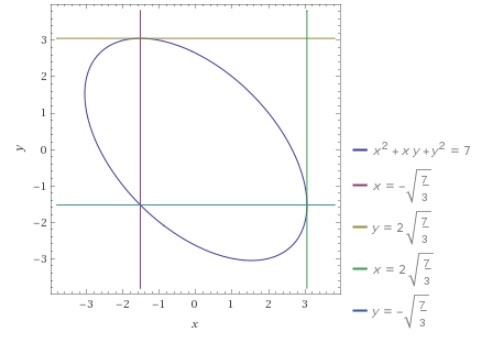
How Do You Find All Points On The Curve X 2 Xy Y 2 7 Where The Tangent Line Is Parallel To The X Axis And The Point Where The Tangent Line

Answered Find All The Second Order Partial Bartleby

2

What Is The Derivative Of 3xy

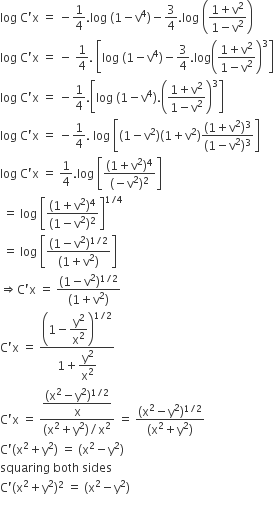
Prove That X2 Y2 C X2 Y2 2 Is The General Solution Of The Differential Equation X3 3xy2 Dx Y3 3x2y Dy Where C Is A Parameter From Class

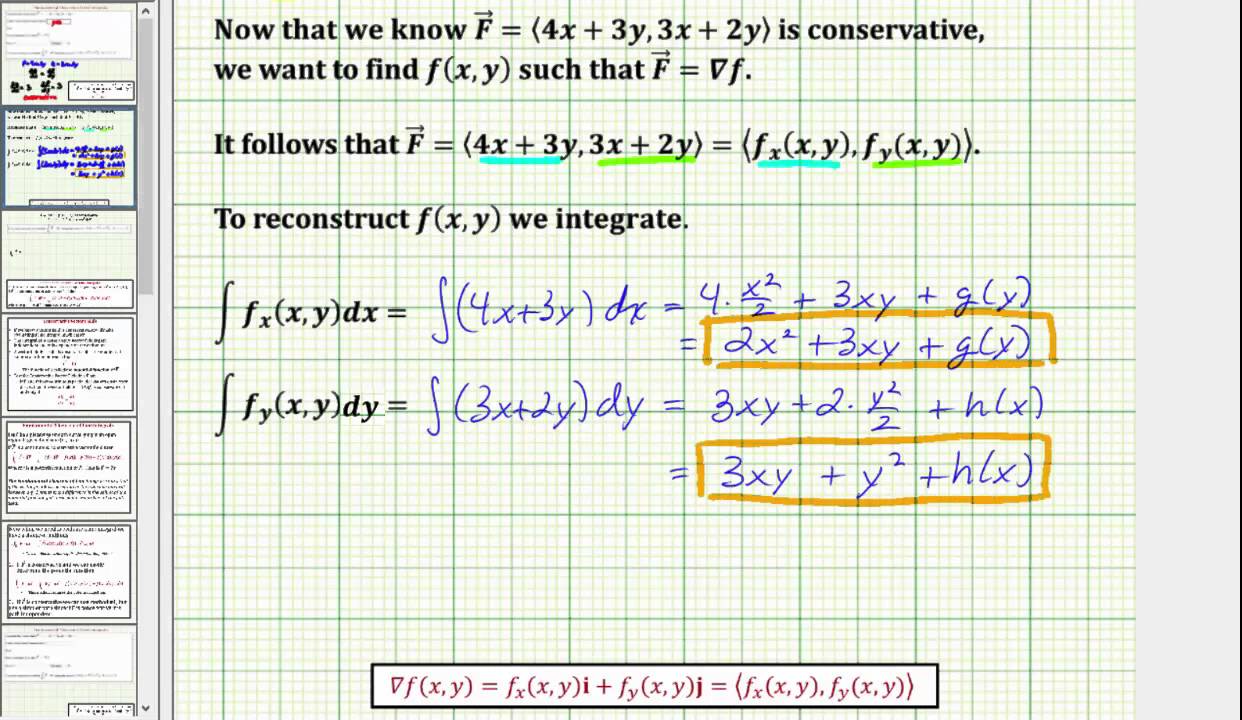
15 3 Line Integrals Over Vector Fields Chapter 15 Vector Analysis Part Calculus Iii

Use Partial Derivatives To Find Dy Dx If Y F X Is Chegg Com

If 4x 2 Xy 3xy Y 2 12 5 Find X 2y 2x Y Brainly In

Dfrac2z 3z4i Dfracpi4 2 Then See How To Solve It At Qanda

If X Y Are Integral Solutions Of 2x 2 3xy 2y 2 7 Then

If U F R Where R 2 X 2 Y 2 Then 2u X 2 2u Y 2

Jacobians New

Find Dydx Where X 2 Y 2 3xy 1

Find All The Partial Derivatives Of The First And The Chegg Com

2

Exercises 1 Show That U X Y Is Harmonic In Some Chegg Com

Doc Partial Derivative Mcq S Assignement Innocentboy Nishant Academia Edu

Solutions To Implicit Differentiation Problems

If X 3 Y 3 3xy 2 3x 2y 1 0 Then At 0 1 Dy Dx Youtube

2

Partial Differentiation

If X 2 Y 2 3xy 0 And X Gt Y Then Find The Value Of Log

2

2

T Qmo3 If Uleft X1yright 4x Y See How To Solve It At Qanda

Pdf Complete Solutions Differential Equations With Modeling Applications Differential Equations With Boundary Value Problems 5th Edition Juan Carlos Becerra Linares Academia Edu

Solving A System Of Equations Using A Matrix Precalculus Socratic

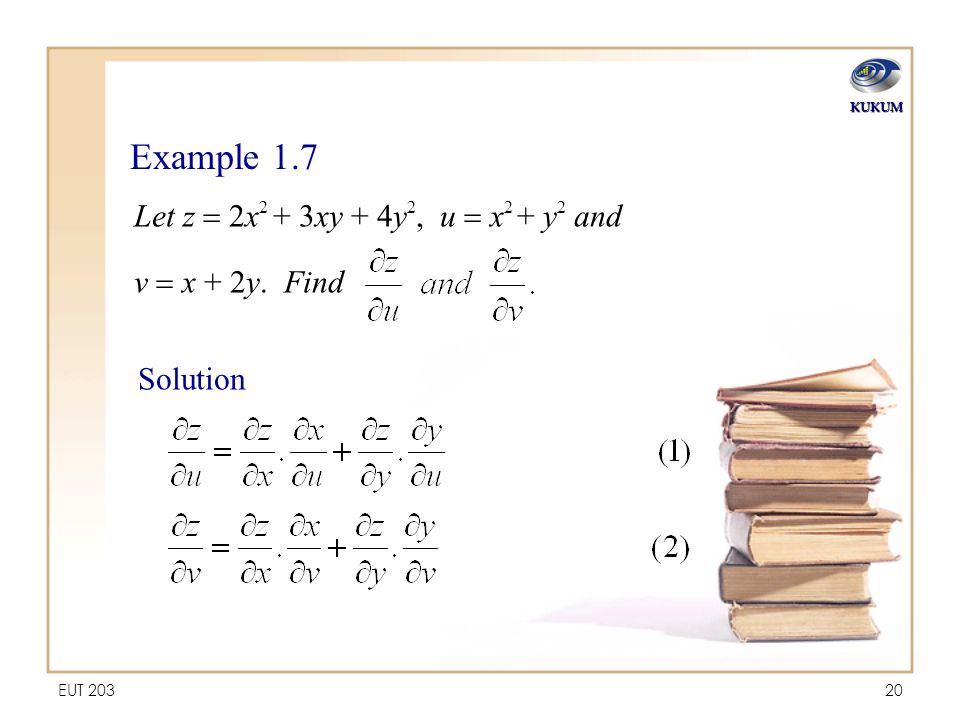
Chapter 1 Partial Differential Equations Ppt Video Online Download

2

How To Solve X 3 3xy 2 Dx 3x 2y Y 3 Dy Quora

2

If Math 3x 4y Sqrt Xy Math How Can You Prove That Math X 2 Y 2 Xy Math Quora